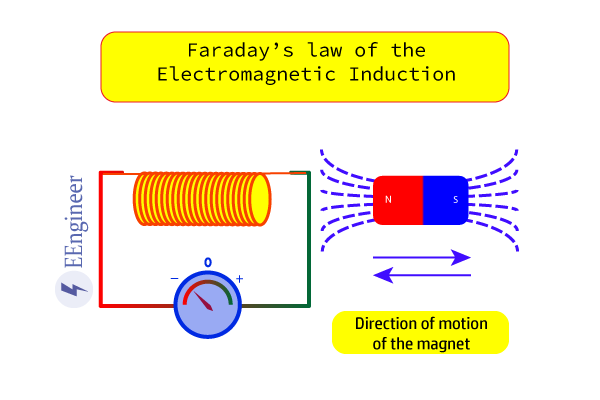
When a conductor cuts a magnetic field, a dynamically induced EMF (voltage) is produced in it. Also, magnitude of induced EMF is directly proportional to the rate of change of flux linkages. So this is called Faraday’s law of Electromagnetic Induction.

• If the flux is time In-varying in nature, additionally it requires relative motion between flux and conductor, for the effective rate of change of flux linkages. Later one should rotate with respect to the other.
• If the flux is time-varying, then it automatically produces voltage with the stationary conductor, because of the inherent rate of change of flux linkages. So there is no need for relative motion.

The formula for Faraday’s law of Electromagnetic Induction (EMI)

Also, λ (flux linkages) are the interactions between flux and conductors.

\lambda=N\times\phi

N= Number~of~conductors

\lambda=flux~linkages

\phi=flux

e=\frac{\partial \lambda}{\partial t}=\frac{\partial (N\phi)}{\partial t}

e=-N\frac{\partial \phi}{\partial t}

Following (-) negative sign explains Lenz’s law. So, this means Induced EMF will oppose the rate of change of flux.

e=\frac{-N*\partial \phi}{\partial t}*\frac{\partial i}{\partial i}

e=\frac{-N*\partial \phi}{\partial i}*\frac{\partial i}{\partial t}

e=-L*\frac{\partial i}{\partial t}

Finally, this is the formula derived from Faraday’s law of electromagnetic induction.

L = Inductance (unit: Henery)

Note: when there is a rate of change of flux linkages, the conductor b